(Cover Artist: Olivia Belin)
Most mathematicians’ careers are dedicated to computation, formulas, and solving problems. A student might need to know the formula for the area of a triangle or the sum of internal angles in a polygon for their upcoming geometry test. Another student might need to know how to use the formula d = rt to solve a word problem about John biking uphill and downhill at different speeds. However, computation is just one side of mathematics; there is another that must be mastered: proofs.
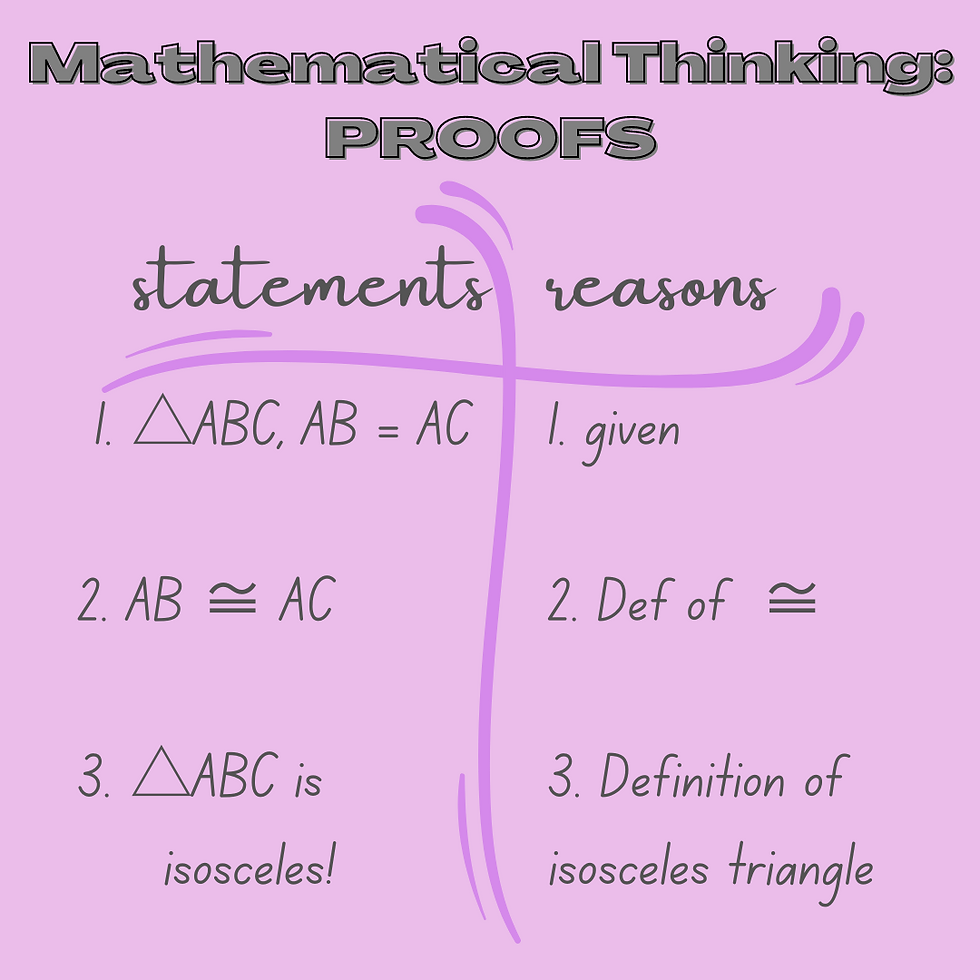
At the heart of most pure mathematics is a focus on how to do proofs, so it’s important to get acquainted with them even if you have a passing interest in pure mathematics. Put simply, proofs are a way to justify the truth of a given mathematical statement using already proved statements. Many people’s first introduction to proofs are two-column proofs, in which the exact statements that lead to the eventual conclusion and reasons for each statement are numbered and listed side-by-side in (you guessed it!) a two-column table. These are typically introduced in a geometry class as a tool for organization, especially since it’s the first class where a lot of theorems are encountered.
However, proofs go much further than their two-column variety. Although their tight structure makes it a good introduction to proof-writing, that same rigidity also means that there’s a lot lacking in a two-column proof. There’s simply not as much freedom or variety with two-column proofs. For example, induction, a fairly well-known method of proof that uses a base case with a hypothesis in order to prove an infinite number of statements, can’t be represented using two-column proofs.
In contrast, paragraph proofs are a lot freer and dynamic, but at the cost of being more difficult to get a hold of and master. But it’s this that makes writing proofs so rewarding. Constructing a paragraph proof, or really any proof, to reach a conclusion makes you really think about how to maneuver yourself into the statement you want to prove. Compared to solving a problem and doing computation, writing a proof is like exercising a different, but related muscle. Solving problems can help you build intuition for writing proofs and writing proofs can help you learn strategies for solving problems.

🤐